Origami models

The instructions for most of the models I built can be found Michał Kosmulski's website. This other website also shows some astonishingly beautiful models.

	Spiked snub dodecahedron with pyramids on pentagonal faces, 210 modules (instructions). The light-green pieces form a Hamilton cycle on the dodecahedron frame, and the pentagonal faces on one side of the Hamilton cycle are colored red and orange, while the faces on the other side are colored dark-blue and light-blue. The model illustrates the famous Four Color Theorem (the faces of any planar graph can be four-colored such that any two neighboring faces receive different colors). For planar graphs that have a Hamilton cycle (as the dodecahedron) the theorem is particularly easy to prove, but otherwise much harder.
	Buckyball, 120 modules (instructions)
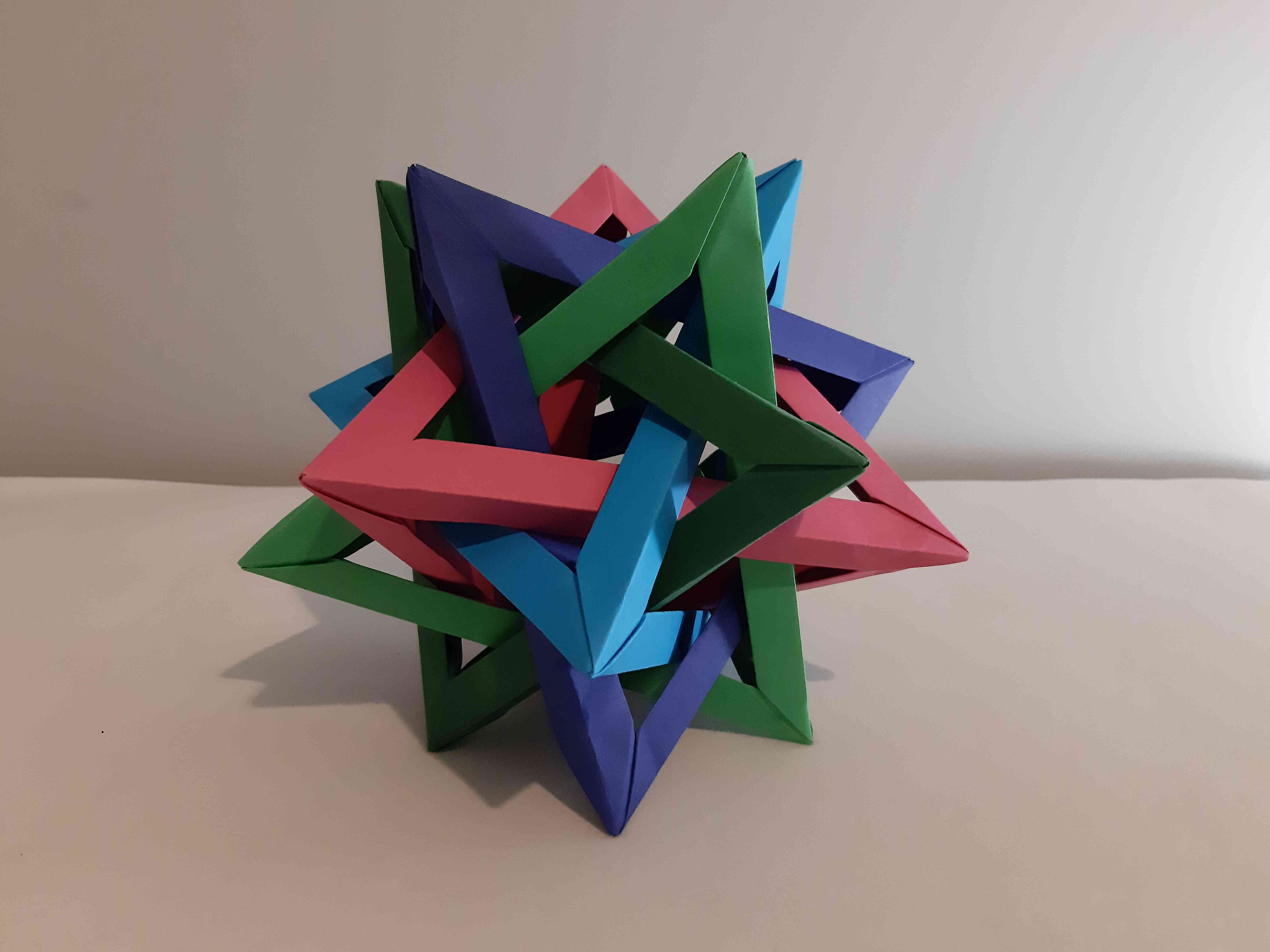
	Five interlocked tetrahedra, 30 modules (instructions)
	Spiked icosahedron, 30 modules
	Three spiked icosahedra, 30 modules each (instructions)
	Cuboctahedron, 20 modules (instructions)
	Ring from interlocked cubes, 192 modules (instructions). This one makes for a nice Christmas decoration. I used packing paper that is colored green on one side and red on the other.
	Group of elephants
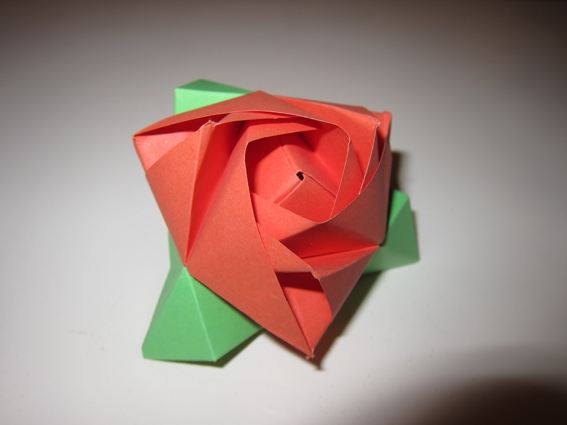
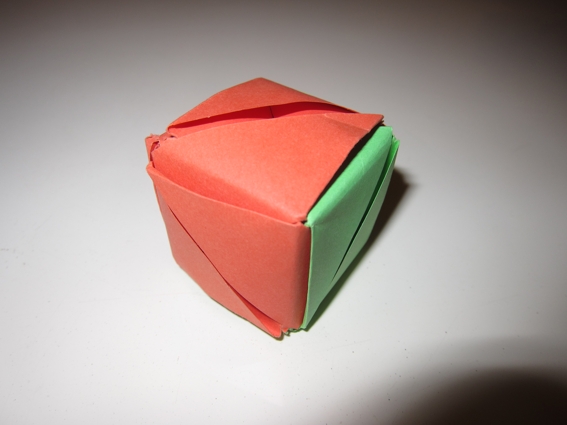
	Unfoldable origami rose cube (instructions). This model I find particularly fascinating. The design is simple and yet fully conveys all the features of a real rose. In addition, the cube/rose transformation is absolutely surprising. Makes for a really beautiful gift.